Research Interest
My primary field of research is Einstein's theory of general relativity, and I specialize in numerical solution of the field equations. Some of my current projects include: understanding the nature of binary compact object (black hole and neutron star) mergers and the gravitational waves emitted during the collision, critical phenomena at the threshold of gravitational collapse, the stability and dynamics of higher dimensional black holes, and the nature of singularities that generically appear in black hole and cosmological spacetimes. The non-linearity and complexity of Einstein's equations make it challenging to solve even numerically, and some portion of my time is spent designing algorithms to efficiently solve the equations in parallel on large computer clusters, and software to manipulate and visualize the simulation results.



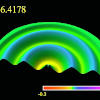

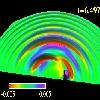

Animations: (a) gravitational waves and (b) lapse function from an equal mass binary black hole merger, (c) apparent horizon embedding diagram of an unstable 5 dimensional black string, (d) spherically symmetric and (e) prolate scalar field critical collapse (in spherical polar coordinates with logarithmic radial and time coordinates), the latter showing what may be an instability of the scalar field threshold solution, (f) complex scalar field critical collapse with angular momentum, prolate initial data, again exhibiting similar unstable behavior as the real field (g) fluid rest-mass density from a black hole-neutron star merger.
Publications: gr-qc listing
Other Affiliations
Associated Faculty in Department of Astrophysical Sciences, Department of Mathematics, and Program in Applied and Computational Mathematics, Princeton University
Distinguished Research Chair, Perimeter Institute
Associate, Canadian Institute for Advanced Research (CIfAR) Cosmology and Gravity Program
Some of the material presented here is based upon work supported in part by the National Science Foundation
